Error-sensitive factors analysis and verification for optical element in-situ measurement device based on phase measuring deflectometry
-
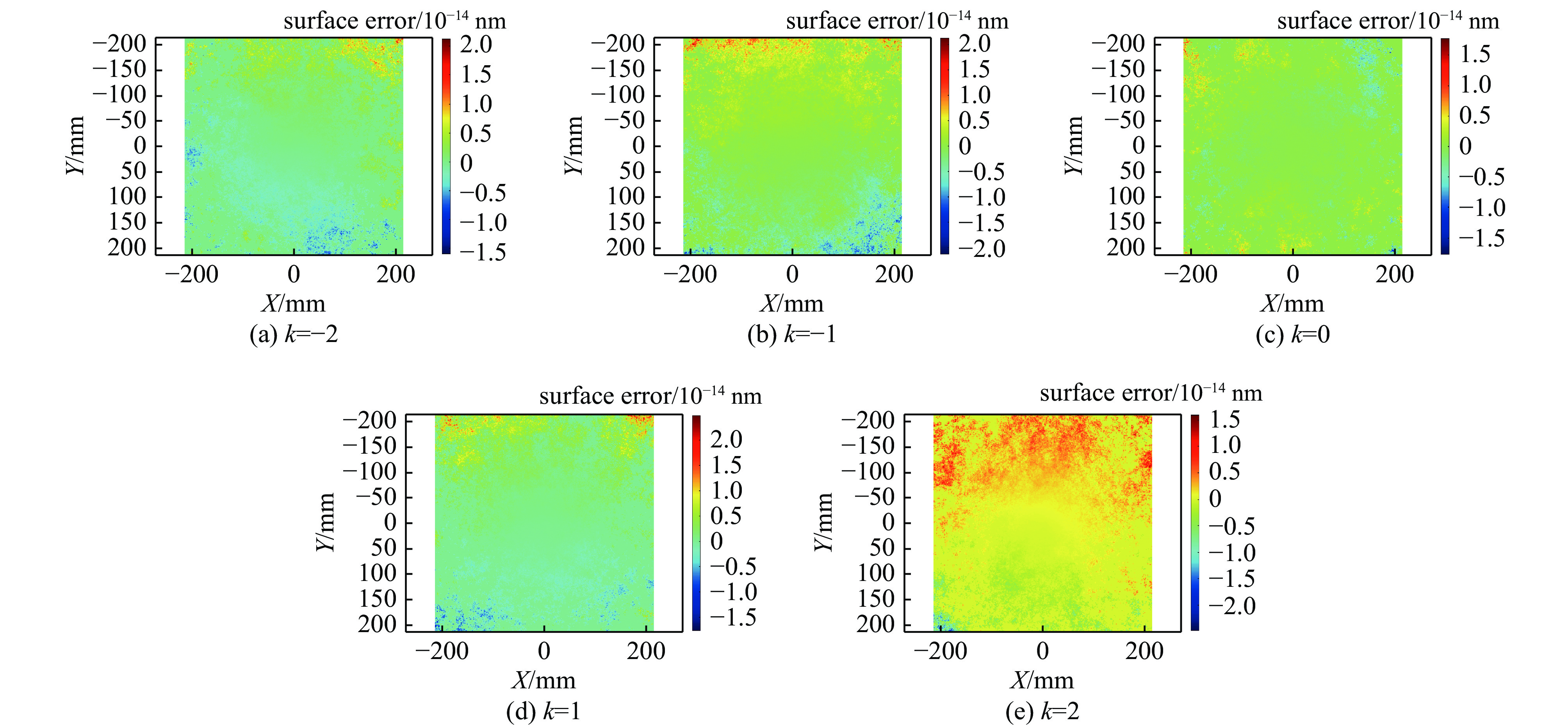
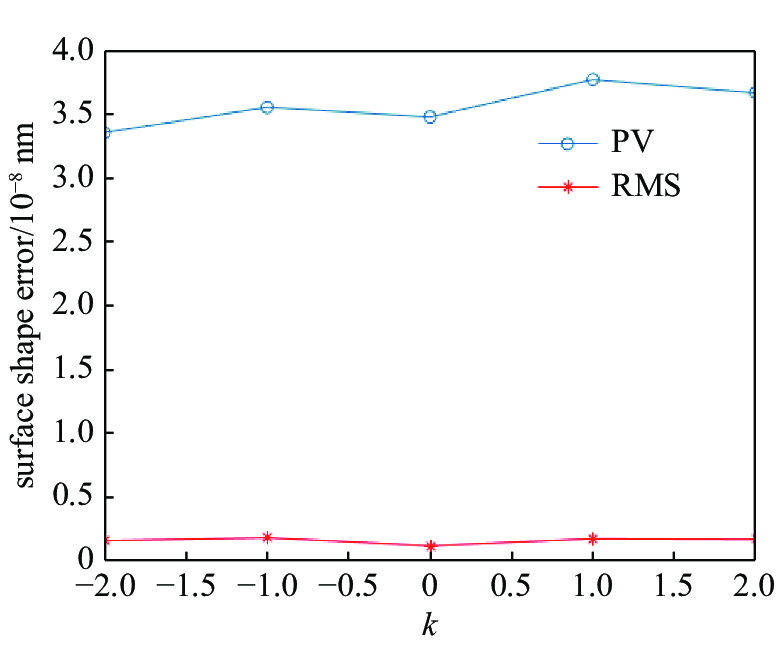
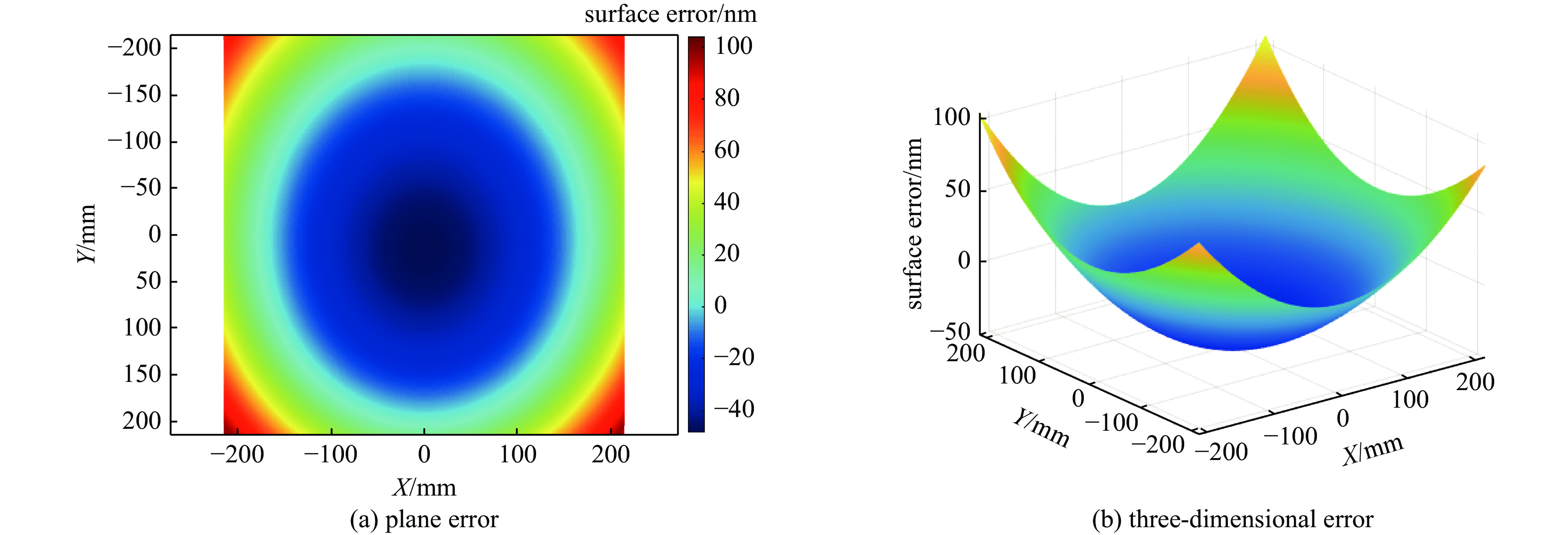
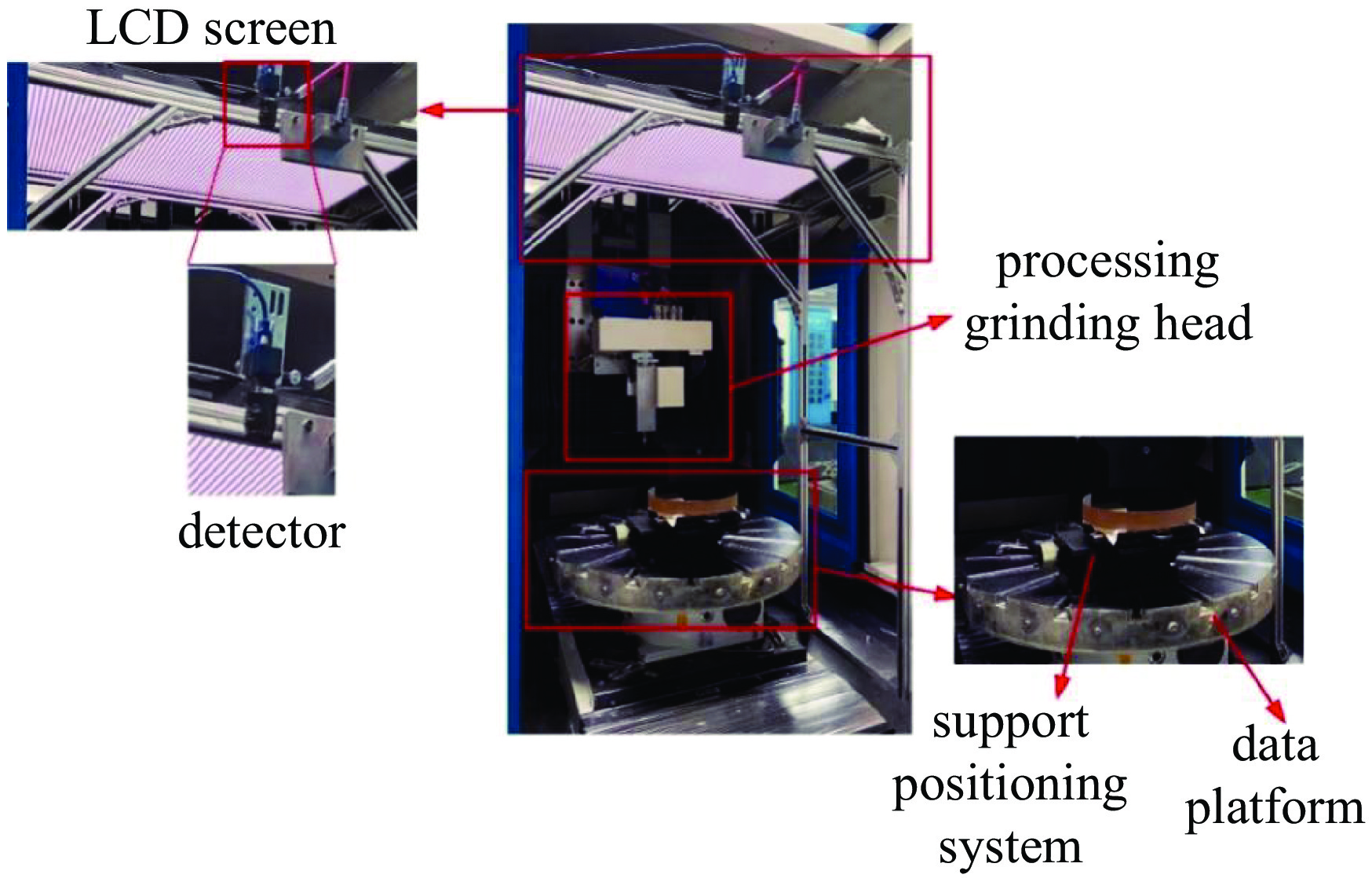
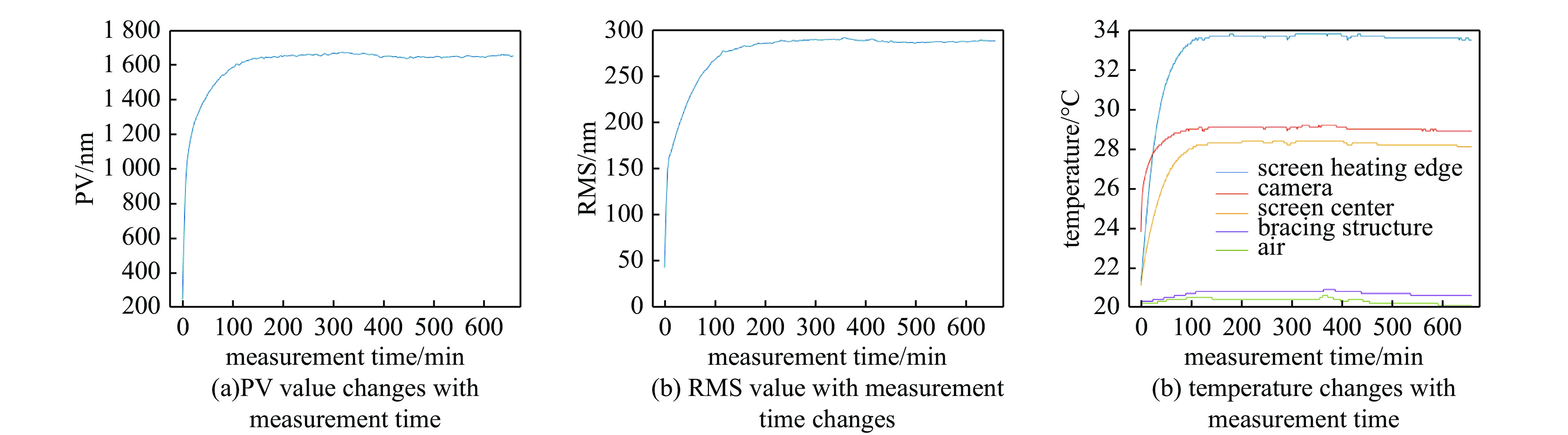
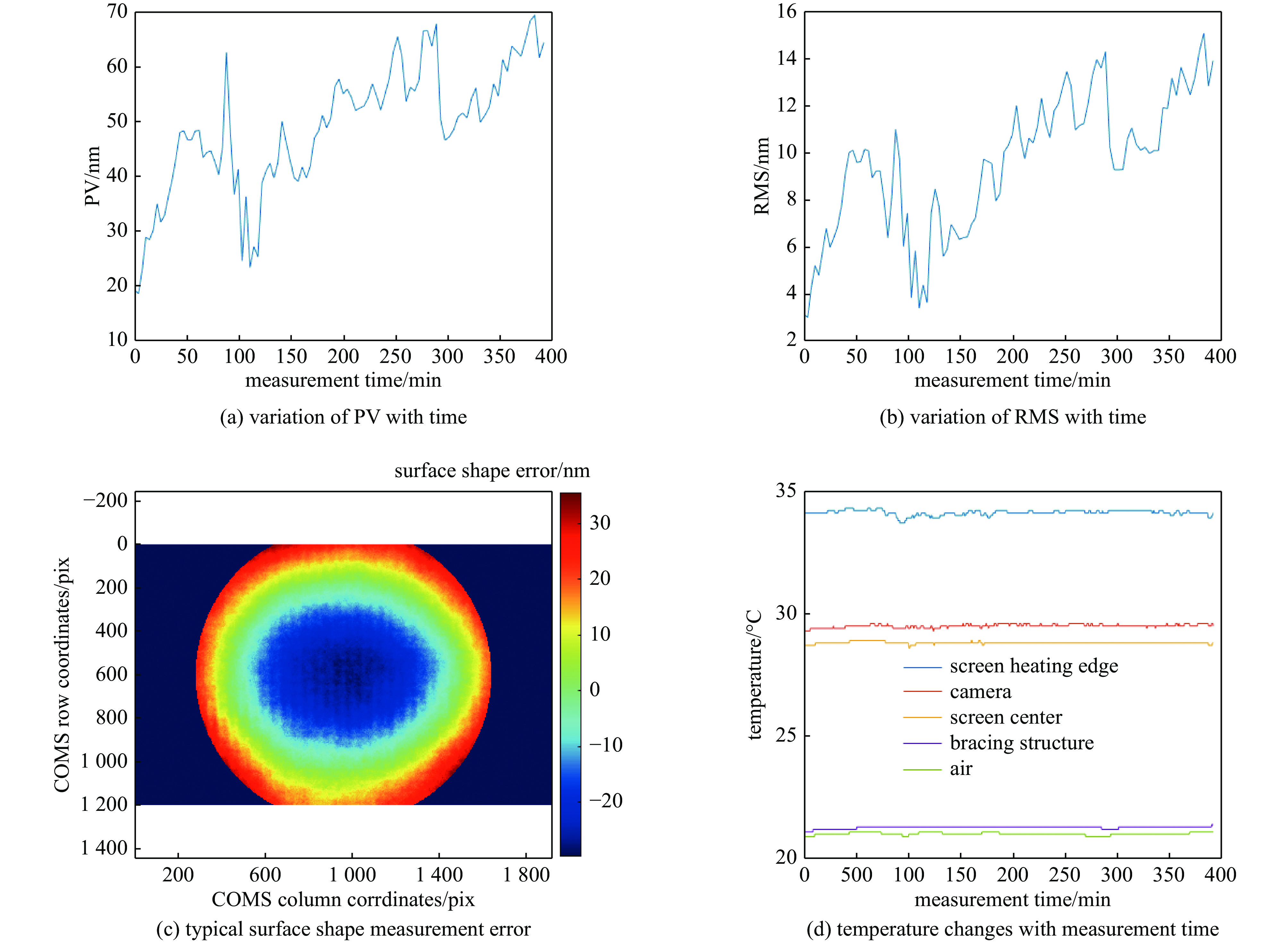
摘要: 针对基于强光元件高精度面形在位检测需求,开展了面形测量误差敏感因素仿真分析,进行了系统结构误差和温度误差对测量结果的影响研究,分析各类误差对测量面形误差的具体影响,设计并搭建在位检测系统,开展系统温度变化、系统重复性、系统稳定性等测量实验。研究结果表明:所建立的逆向哈特曼仿真检测模型可用于平面、球面、非球面、自由曲面等各类型被测面,各类影响因素对测量结果的影响主要体现在低频误差上,对高频误差的影响相对较小,搭建的在位检测系统6 h内测量面形误差PV值最大不超过68 nm(约λ/10),RMS值最大不超过15 nm(约λ/40)。Abstract: Based on optical element’s high precision in-situ measurement requirements, this paper carries out the sensitive factor simulation analysis, studies the influence of systematic structural errors and temperature errors on the measurement results, and designs and builds an in-situ measurement device to carry out measurement experiments of system temperature change, system repeatability and system stability. The results show that the simulation detection model can be used for plane/spherical/aspherical/free surface, the influence on the measurement results is mainly reflected in the low frequency error, the high frequency error is relatively small, the maximum PV value of the measurement surface shape error does not exceed 68nm (about λ/10), and the maximum RMS value does not exceed 15 nm (about λ/40).
-
Key words:
- shape measurement /
- in-situ measurement /
- optical element /
- error analysis /
- simulation model
-
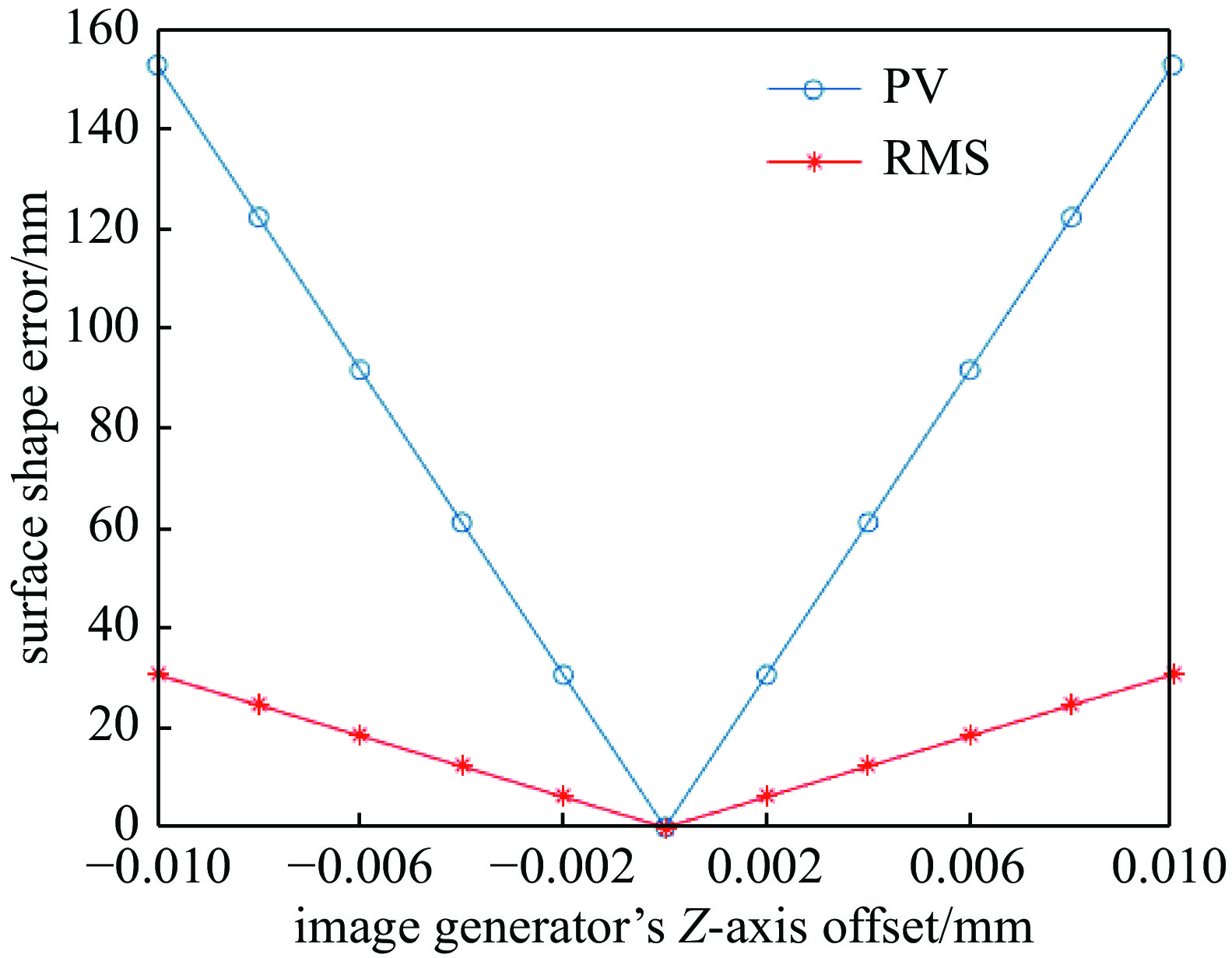
表 1 图像发生器定位误差对测量结果影响
Table 1. Influence of image generator’s positioning error on the measurement results
direction maximum surface shape error/nm corresponding to Zernike coefficients PV RMS number of terms number of main items main types along the X axis 77.2 10.1 2、5、8、15 5 45° primary astigmatism along the Y axis 88.9 18.1 3、4、6、7、9 4、6 defocusing and 0° primary astigmatism along the Z axis 152.7 30.6 3、4、6、7 4、6 defocusing and 0° primary astigmatism 表 2 针孔光阑定位误差对测量结果影响
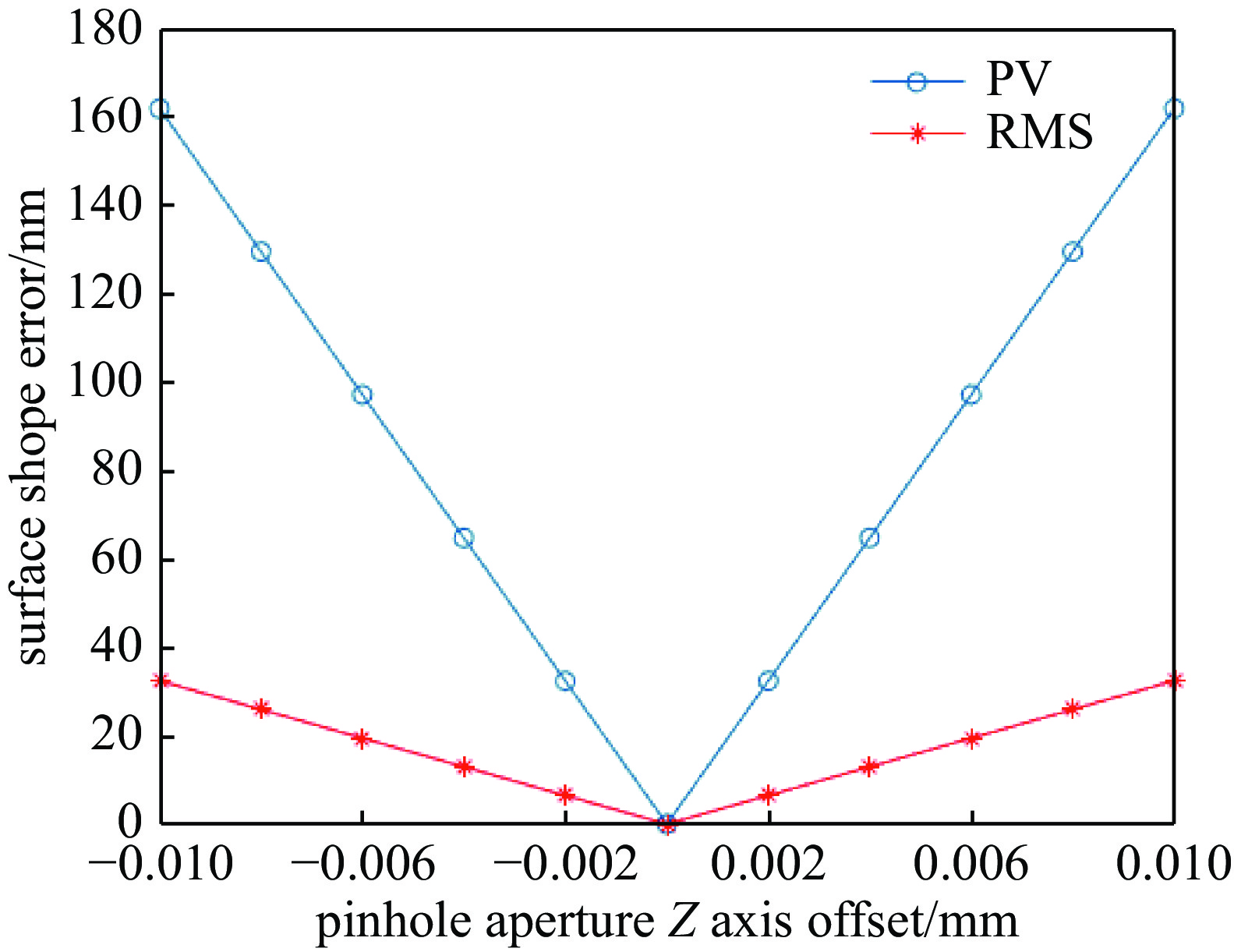
Table 2. Effect of pinhole diaphragm positioning error on measurement results
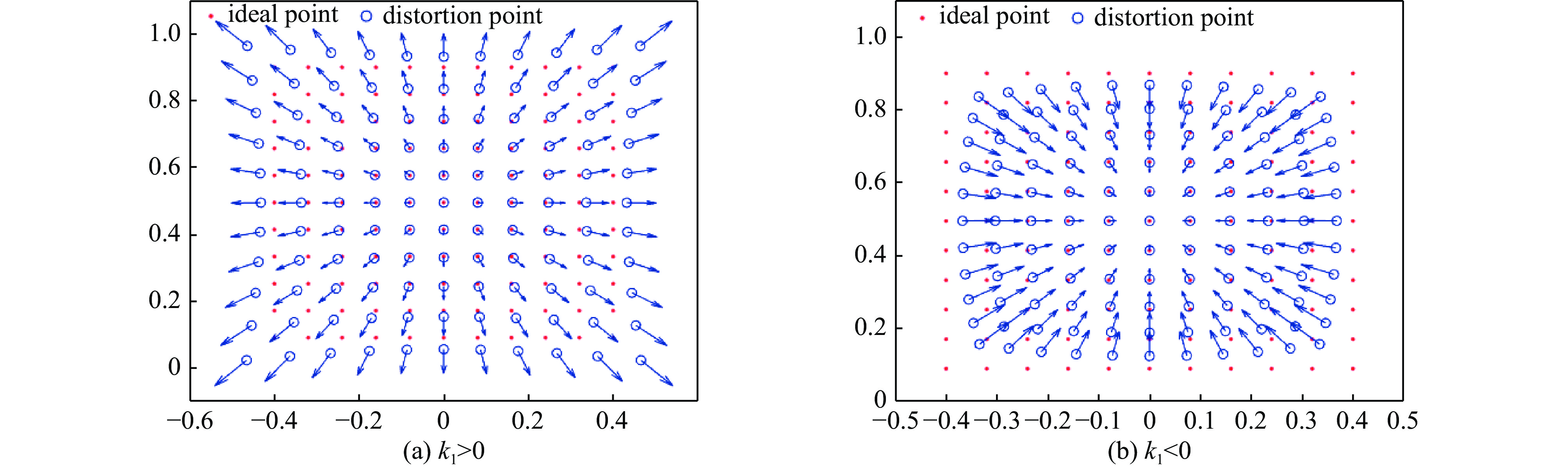
direction maximum surface shape error/nm corresponding to Zernike coefficients PV RMS number of terms number of main items main types along the X axis 81.8 10.8 2、5、8、15 5 45° primary astigmatism along the Y axis 94.2 19.1 3、4、6、7、9 4、6 defocusing and 0° primary astigmatism along the Z axis 161.9 32.4 3、4、6、7 4、6 defocusing and 0° primary astigmatism 表 3 探测器镜头畸变对测量结果影响
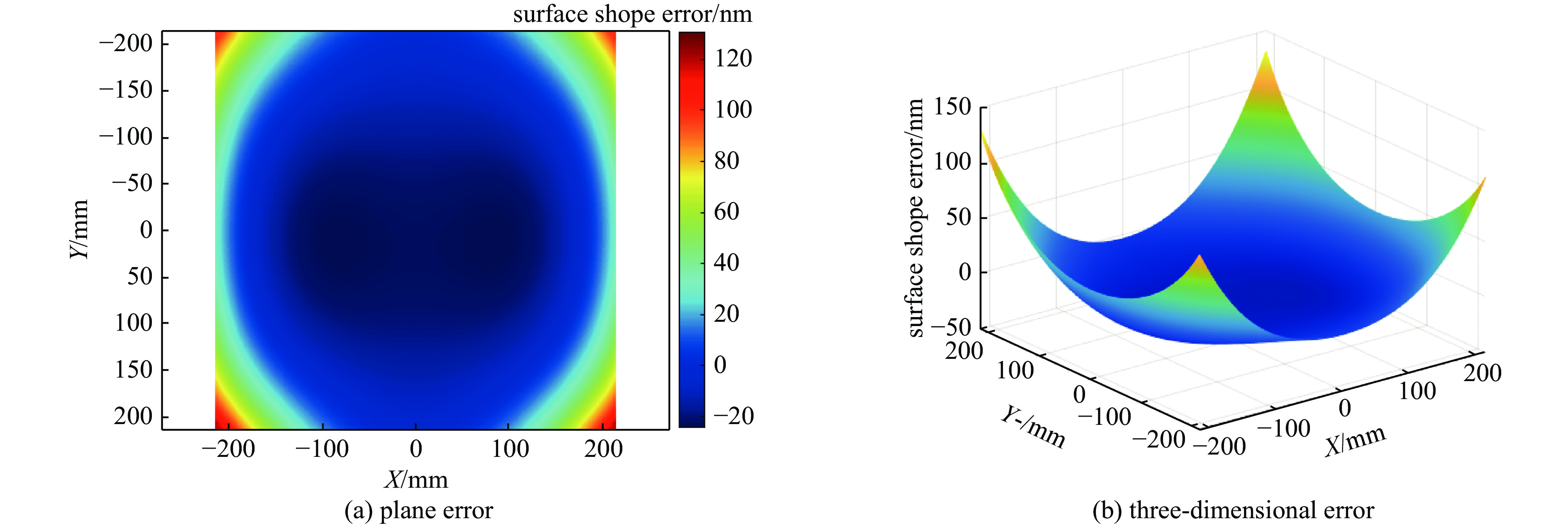
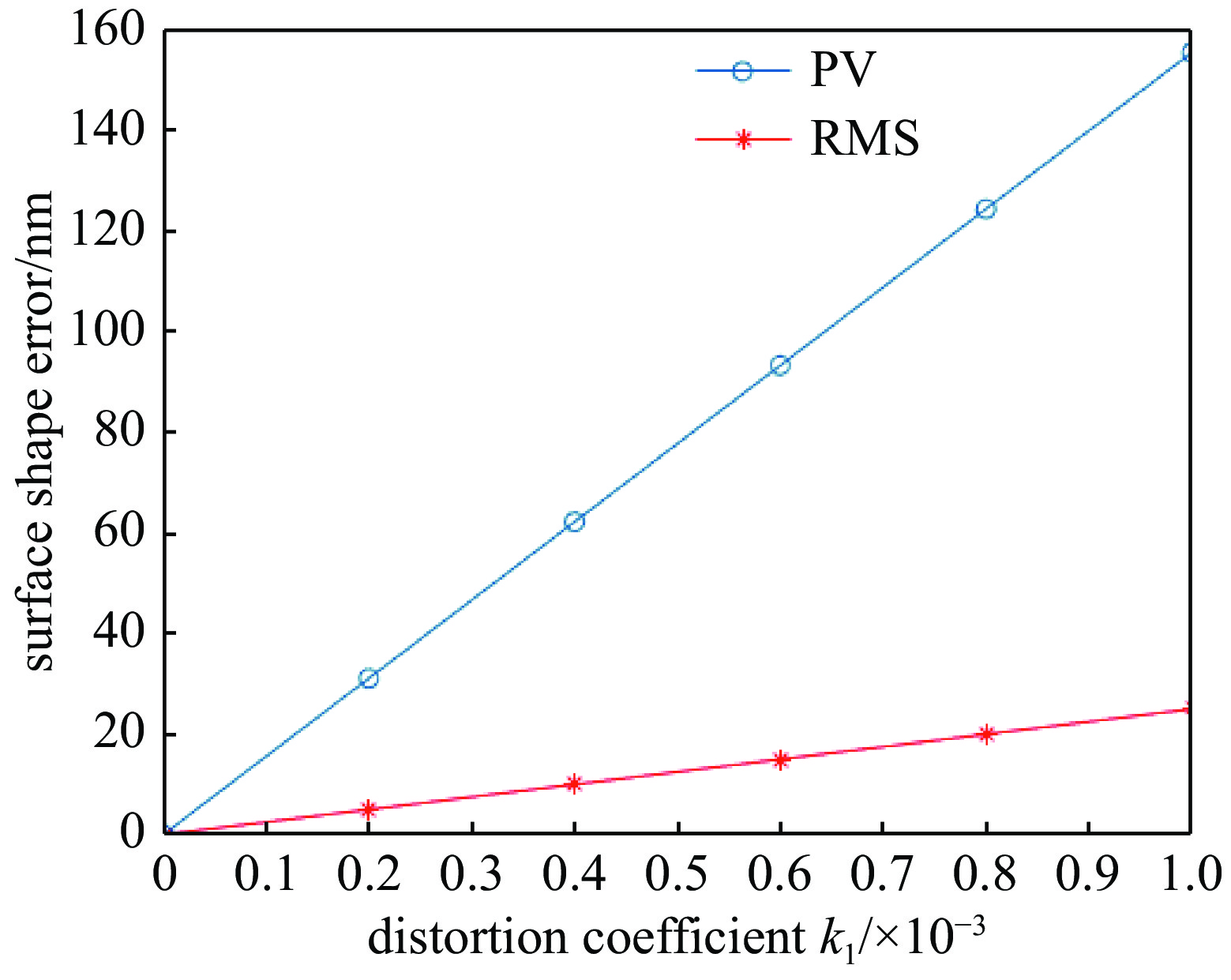
Table 3. Effect of detector lens distortion on measurement results
influencing factor maximum surface
shape error/nmcorresponding to Zernike
coefficientsPV RMS number of
termsnumber of
main itemsmain
typesdetector lens
distortion
(|k1|=1×10−3)pin-cushion distortion 155.3 24.9 3、4、6、11、12、28 4、6、11、12 defocusing, 0° primary astigmatism
and higher-order aberrationsbarrel distortion 155.3 24.9 3、4、6、11、12、28 4、6、11、12 defocusing, 0° primary astigmatism
and higher-order aberrations表 4 温度变化对测量结果影响
Table 4. Effect of temperature change on measurement results
influencing factor maximum surface shape error/nm corresponding to Zernike coefficients PV RMS number of
termsnumber of
main itemsmain types image generator expansion 416.9 85.3 3、4、5、6、7 3、4、5 defocusing, 45° primary astigmatism,
0° primary astigmatismdetector expansion 99.7 20.4 3、4、6、7 4 focusing out support structure expansion 4.9 1.0 3、4、6、7、9 4、6 defocusing and 0°
primary astigmatism表 5 检测系统参数
Table 5. Detection system parameters
measurement serial number PV/nm RMS/nm measurement serial number PV/nm RMS/nm 1 19.2 3.1 16 46.6 9.6 2 18.4 2.9 17 46.7 9.6 3 21.7 4.0 18 47.7 10.0 4 28.3 5.4 19 49.3 10.3 5 29.7 4.8 20 44.9 9.3 6 27.7 5.2 21 42.6 8.8 7 33.7 6.6 22 45.5 9.5 8 34.4 6.6 23 43.5 8.7 9 31.3 5.9 24 41.7 7.3 10 34.3 6.6 25 41.3 6.6 11 37.4 7.1 26 50.1 9.0 12 40.5 8.1 27 60.6 10.9 13 44.5 9.3 28 45.7 9.0 14 48.2 10.0 29 37.6 6.3 15 48.1 10.1 30 39.9 7.1 -
[1] 张梦瑶, 田爱玲, 王大森, 等. 基于逆向优化策略的面形绝对检测平移量研究[J]. 中国激光, 2022, 49:1804003 doi: 10.3788/CJL202249.1804003Zhang Mengyao, Tian Ailing, Wang Dasen, et al. Translation of surface shape absolute testing based on reverse optimization strategy[J]. Chinese Journal of Lasers, 2022, 49: 1804003 doi: 10.3788/CJL202249.1804003 [2] 侯溪, 张帅, 胡小川, 等. 超高精度面形干涉检测技术进展[J]. 光电工程, 2020, 47:200209Hou Xi, Zhang Shuai, Hu Xiaochuan, et al. The research progress of surface interferometric measurement with higher accuracy[J]. Opto-Electronic Engineering, 2020, 47: 200209 [3] Ye Meitu, Liang Jin, Li Leigang, et al. Simultaneous measurement of external and internal surface shape and deformation based on photogrammetry and stereo-DIC[J]. Optics and Lasers in Engineering, 2022, 158: 107179. doi: 10.1016/j.optlaseng.2022.107179 [4] Su Peng, Wang Yuhao, Burge J H, et al. Non-null full field X-ray mirror metrology using SCOTS: a reflection deflectometry approach[J]. Optics Express, 2012, 20(11): 12393-12406. doi: 10.1364/OE.20.012393 [5] Berger G, Petter J. Non-contact metrology of aspheric surfaces based on MWLI technology[C]//Proceedings of SPIE 8884, Optifab 2013. 2013: 88840V. [6] Anderson D S, Burge J H. Swing-arm profilometry of aspherics[C]//Proceedings of SPIE 2536, Optical Manufacturing and Testing. 1995: 169-179. [7] Wan Xinjun, Bin Boyi, Xie Shuping, et al. Development of an integrated freeform optics measurement system based on phase measuring deflectometry[C]//Proceedings of SPIE 10847, Optical Precision Manufacturing, Testing, and Applications. 2018: 1084710. [8] Guo Chunfeng, Hu Anduo. Three-dimensional shape measurement of aspheric mirrors with null phase measuring deflectometry[J]. Optical Engineering, 2019, 58: 104102. [9] Chaudhuri R, Papa J, Rolland J P. System design of a single-shot reconfigurable null test using a spatial light modulator for freeform metrology[J]. Optics Letters, 2019, 44(8): 2000-2003. doi: 10.1364/OL.44.002000 [10] Fang Fengzhou, Zhang Xiaodong, Weckenmann A, et al. Manufacturing and measurement of freeform optics[J]. CIRP Annals, 2013, 62(2): 823-846. doi: 10.1016/j.cirp.2013.05.003 [11] Lei Huang, Idir M, Zuo Chao, et al. Comparison of two-dimensional integration methods for shape reconstruction from gradient data[J]. Optics and Lasers in Engineering, 2015, 64: 1-11. doi: 10.1016/j.optlaseng.2014.07.002 [12] Xu Yongjia, Gao Feng, Jiang Xiangqian. Enhancement of measurement accuracy of optical stereo deflectometry based on imaging model analysis[J]. Optics and Lasers in Engineering, 2018, 111: 1-7. doi: 10.1016/j.optlaseng.2018.07.007 [13] Huang Lei, Ng C, Asundi A K. Dynamic 3D measurement for specular reflecting surface with monoscopic fringe reflection deflectometry[C]//Proceedings of the Computational Optical Sensing and Imaging 2011. 2011: CWC3. [14] Song Lei, Yue Huimin, Kim H, et al. A study on carrier phase distortion in phase measuring deflectometry with non-telecentric imaging[J]. Optics Express, 2012, 20(22): 24505-24515. doi: 10.1364/OE.20.024505 [15] 阮一郎, 李大海, 余林治, 等. 基于相位测量偏折术的成像透镜轴外点波像差测量[J]. 中国激光, 2022, 49:2104003 doi: 10.3788/CJL202249.2104003Ruan Yilang, Li Dahai, Yu Linzhi, et al. Off-axis point wave aberration testing for imaging lens based on phase measuring deflectometry[J]. Chinese Journal of Lasers, 2022, 49: 2104003 doi: 10.3788/CJL202249.2104003 [16] 陈贞屹, 赵文川, 张启灿, 等. 基于立体相位测量偏折术的预应力薄镜面形检测[J]. 光电工程, 2020, 47:190435Chen Zhenyi, Zhao Wenchuan, Zhang Qican, et al. Shape measurement of stressed mirror based on stereoscopic phase measuring deflectometry[J]. Opto-Electronic Engineering, 2020, 47: 190435 [17] Su Peng, Khreishi M, Huang Run, et al. Precision aspheric optics testing with SCOTS: a deflectometry approach[C]//Proceedings of SPIE 8788, Optical Measurement Systems for Industrial Inspection VIII. 2013: 87881E. [18] Faber C, Olesch E, Krobot R, et al. Deflectometry challenges interferometry: the competition gets tougher![C]//Proceedings of SPIE 8493, Interferometry XVI: Techniques and Analysis. 2012: 84930R. [19] 冯婕, 白瑜, 邢廷文. Zernike多项式波面拟合精度研究[J]. 光电技术应用, 2011, 26(2):31-34 doi: 10.3969/j.issn.1673-1255.2011.02.009Feng Jie, Bai Yu, Xing Tingwen. Fitting accuracy of wavefront using Zernike polynomials[J]. Electro-Optic Technology Application, 2011, 26(2): 31-34 doi: 10.3969/j.issn.1673-1255.2011.02.009 -




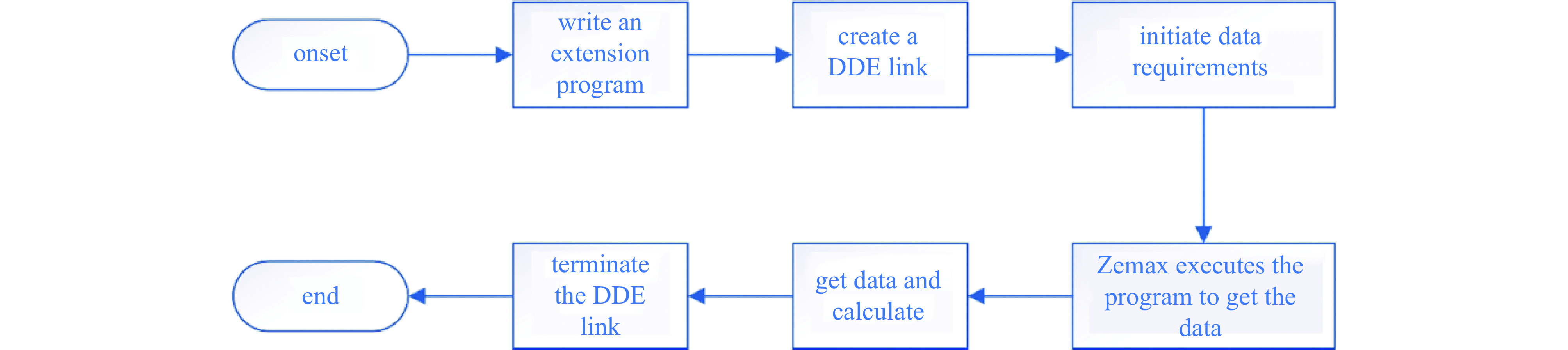
 下载:
下载: