Research on Monte Carlo calculation method for photon absorbed dose
-
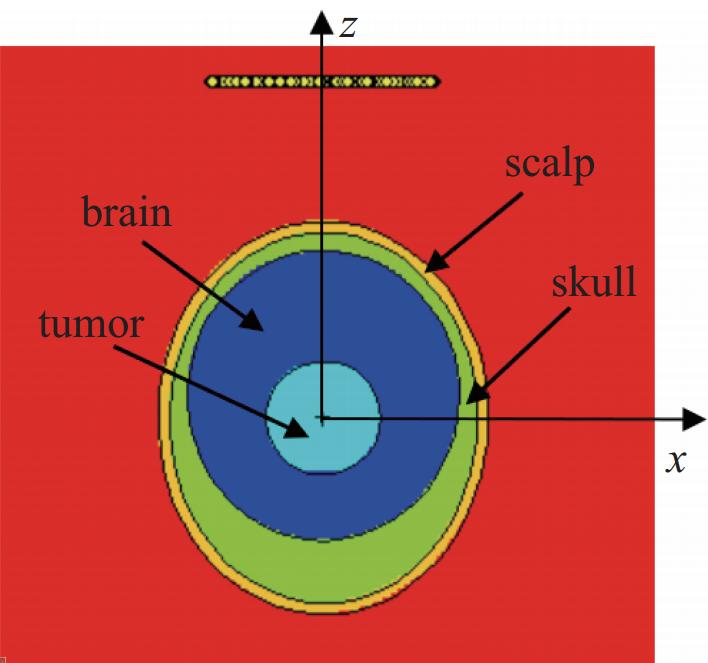
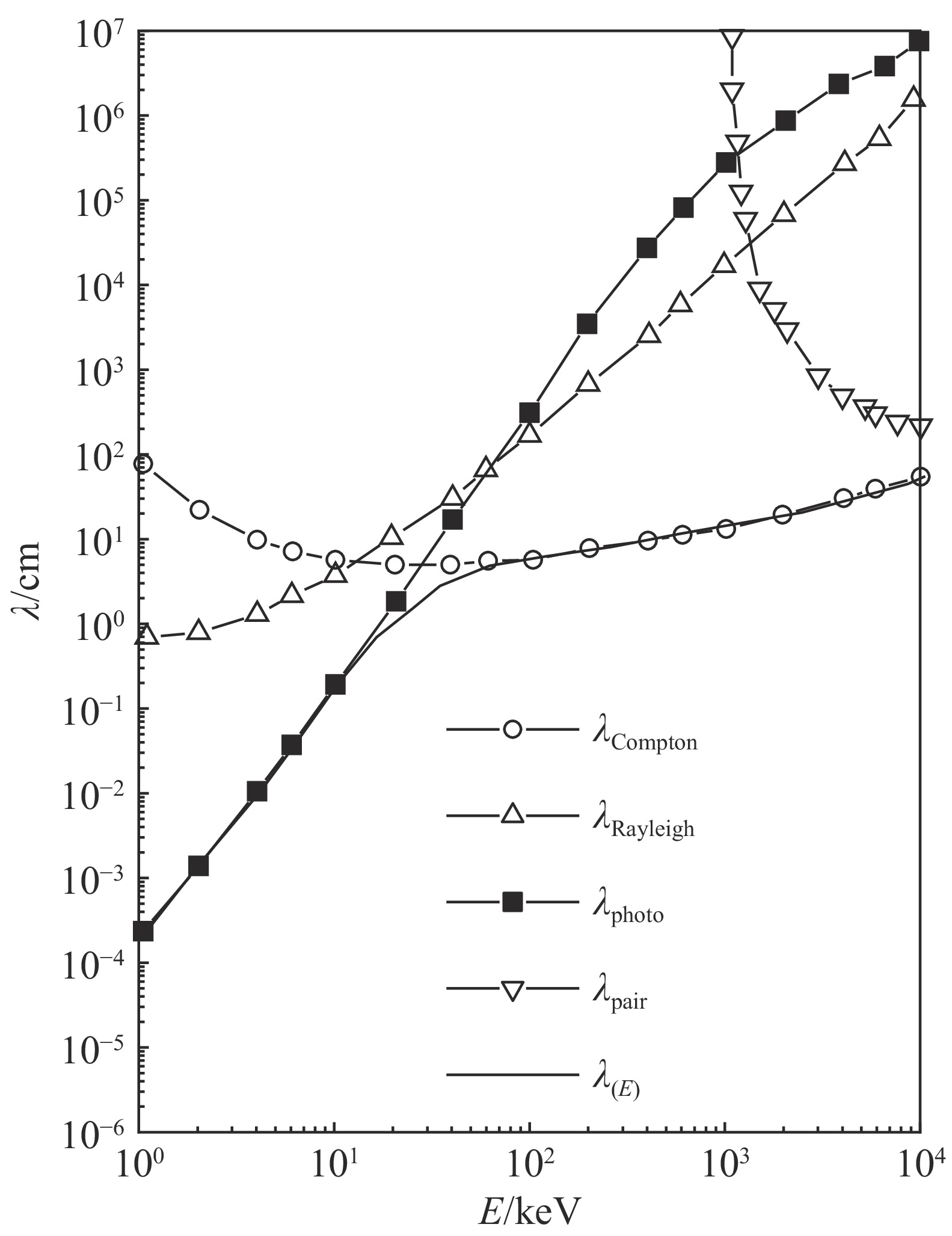
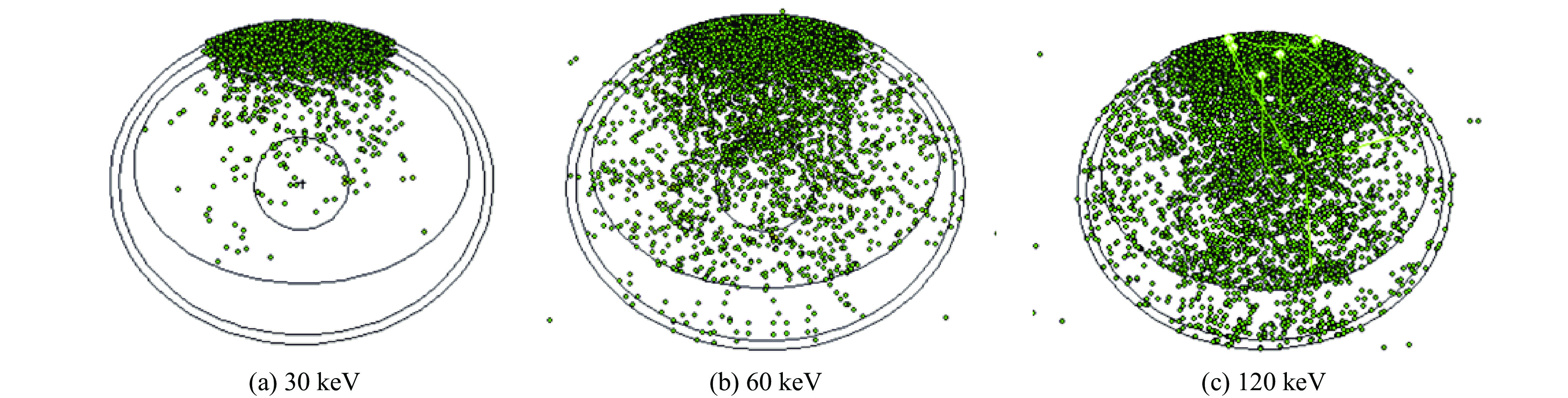
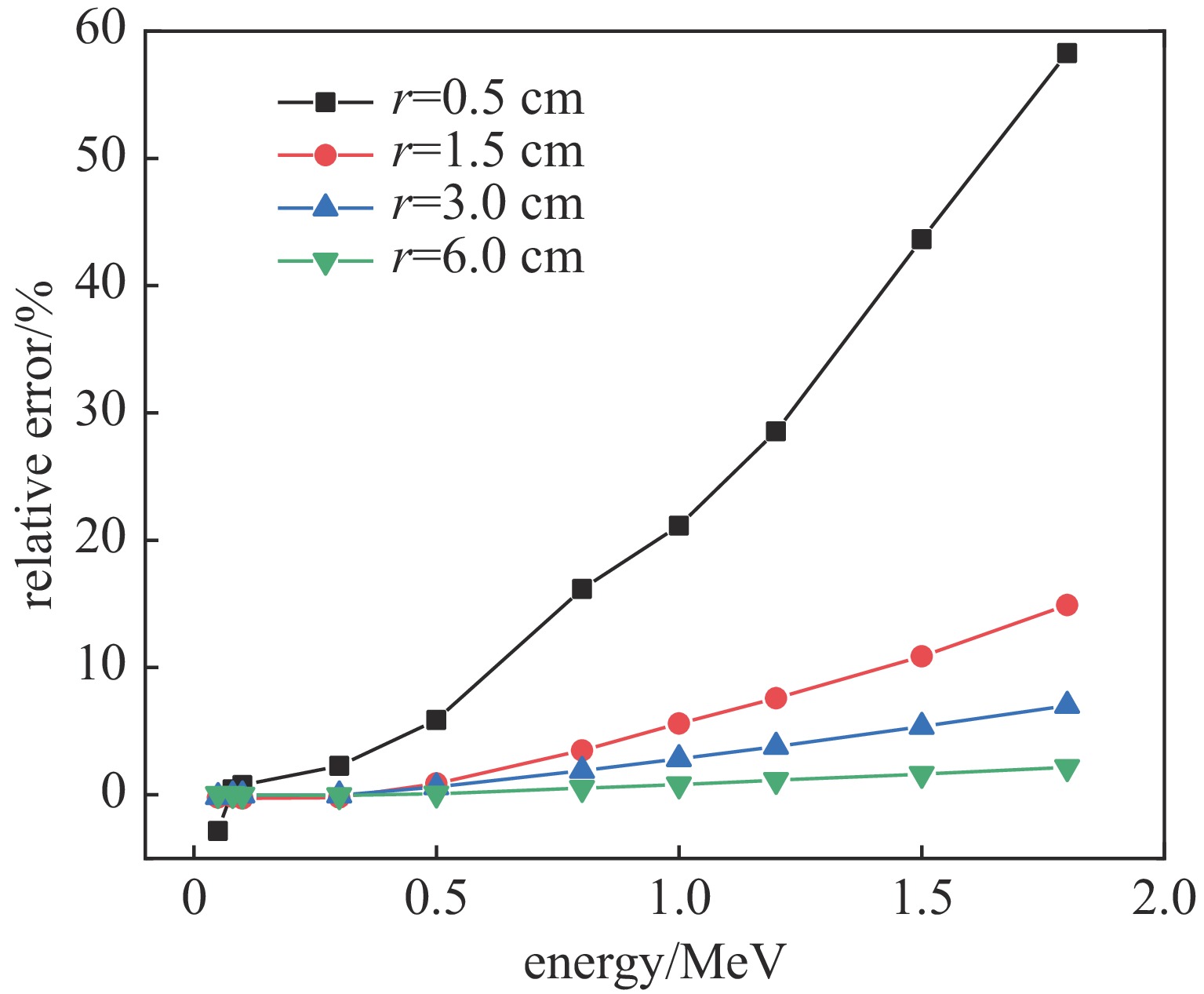
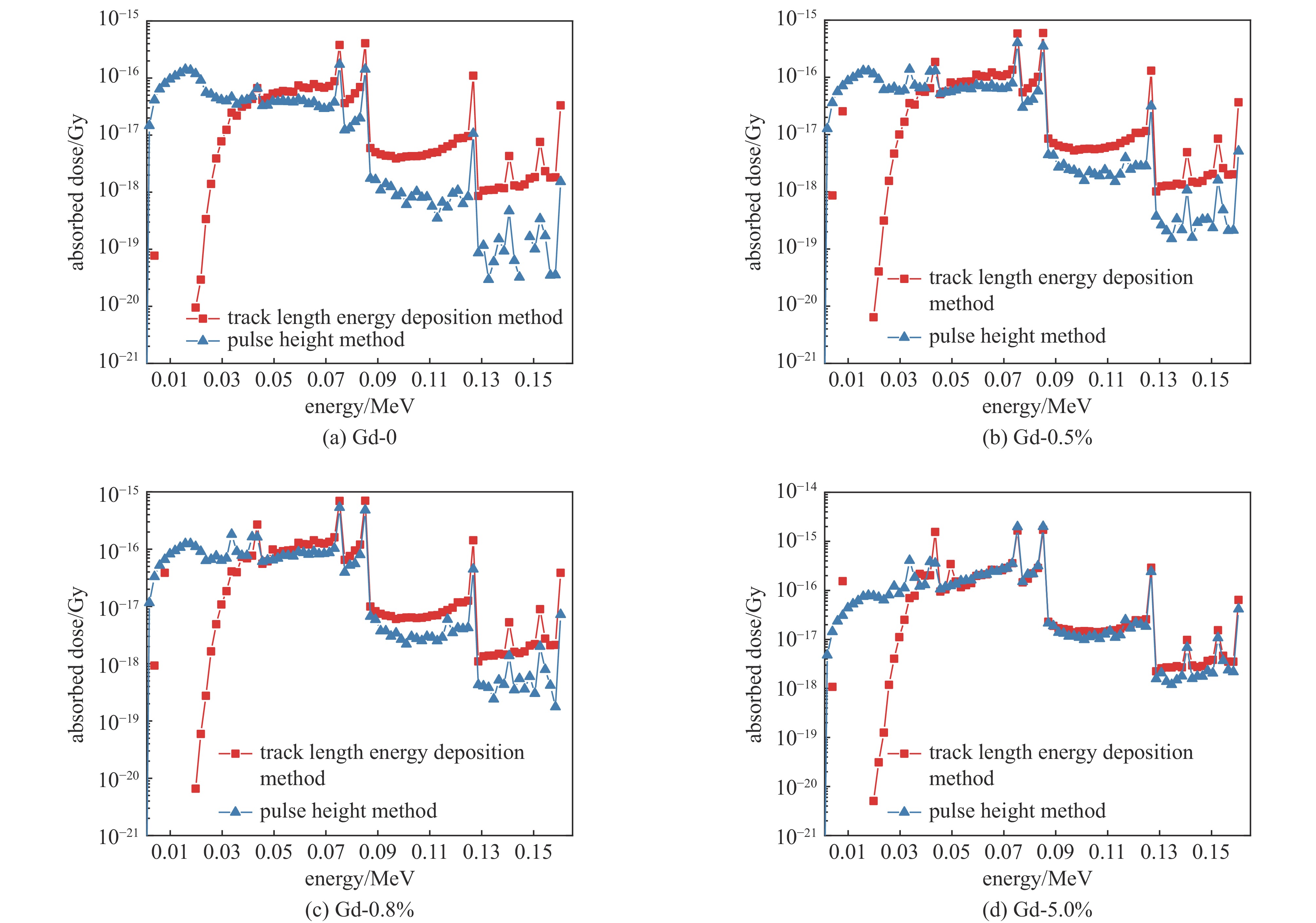
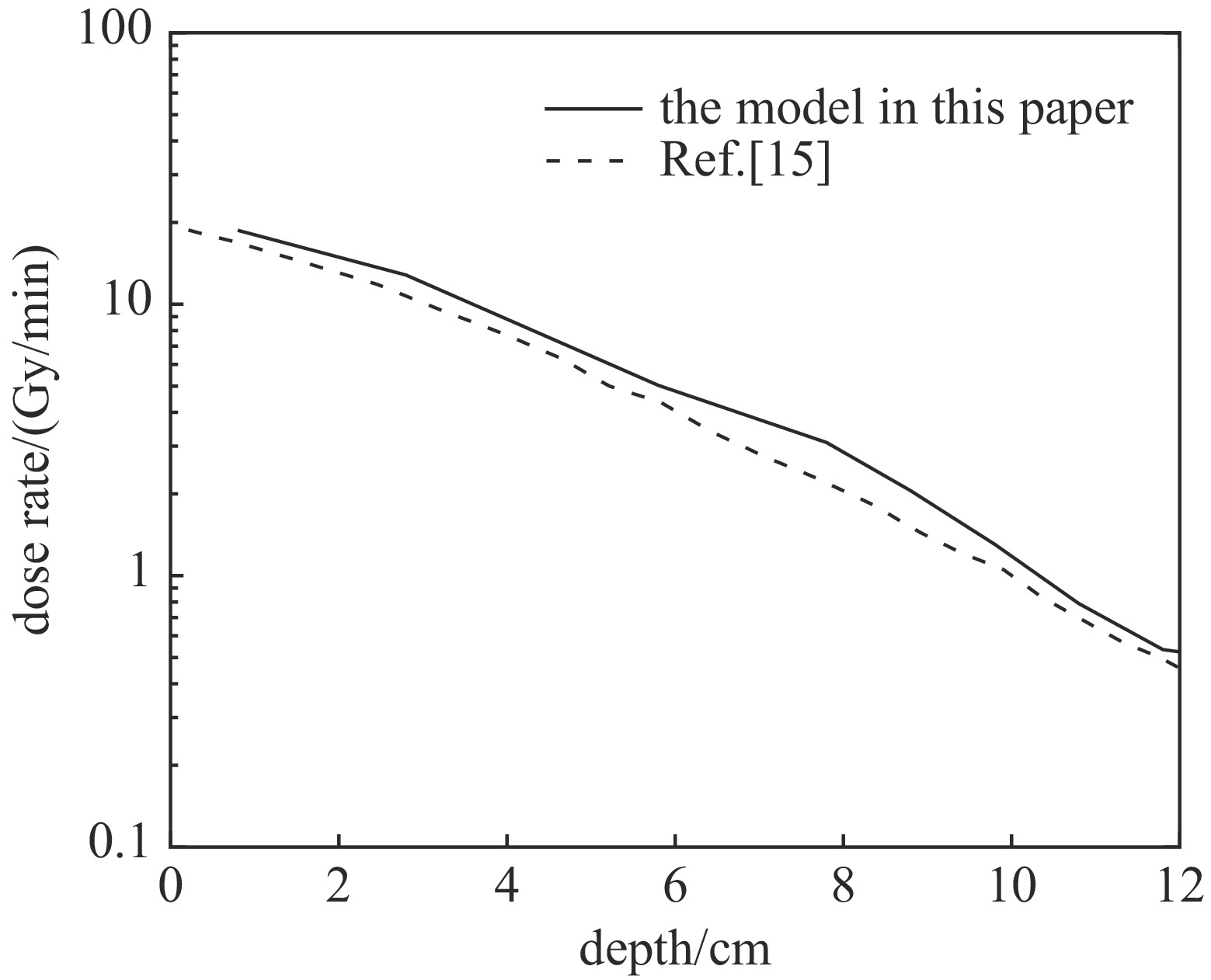
摘要: 为了能够通过选择合适的计算方法对不同辐射情况的光子剂量进行准确有效的计算和评估，对比研究了蒙特卡罗模拟软件中常用的四种光子剂量计算方法：剂量转换系数法、发热数法、径迹长度能量沉积法和脉冲高度计数法，从计算原理出发结合仿真结果分析比较了这四种方法。通过模拟不同能量的单能光子束入射不同体积大小的水球，分析了用比释动能近似吸收剂量导致的误差，并且模拟分析了不同含量的高原子序数元素钆对于吸收剂量计算结果差异的影响。由于转换系数是根据参考人模计算得到的，因此剂量转换系数法只能对吸收剂量进行快速的估计计算，很难在特定情况下得到精准剂量；当高能量光子入射小体积物质时，比释动能会大于吸收剂量，使用发热数法和径迹长度能量沉积法会产生误差，采用脉冲高度计数法更加合适；在低能量和大体积时可以根据计算精度和计算机资源选择任意方法；当组成物质的高原子序数元素含量增多时，径迹长度能量沉积法和脉冲高度计数法之间的计算误差会减少。Abstract: In order to accurately and effectively calculate and evaluate photon doses under different radiation conditions by selecting appropriate calculation methods, this paper compares and studies four commonly used photon dose calculation methods in Monte Carlo simulation software: dose conversion coefficient method, heat number method, track length energy deposition method and pulse height method. Starting from the calculation principle and combining simulation results, these four methods are analyzed and compared. By simulating single energy photon beams with different energies incident on water spheres of different volume sizes, the error caused by approximating absorbed dose with kerma was analyzed, and the influence of different contents of high atomic number element gadolinium in absorbed dose calculation results was simulated and analyzed. Due to the fact that the conversion coefficient is calculated based on the reference human model, the dose conversion coefficient method can only quickly estimate and calculate the absorbed dose, it is difficult to obtain accurate dose in specific situations. When high-energy photons are incident on a small volume of material, kerma will be greater than the absorbed dose, so the heat number method and the track length energy deposition method will produce errors, the pulse height counting method is more suitable. At low energy and large volume, any method can be selected based on computational accuracy and computer resources. When the content of high atomic number elements in a substance increases, the calculation error between the track length energy deposition method and the pulse height method will decrease.
-
Key words:
- photons /
- absorbed dose /
- Monte Carlo method /
- human phantom simulation
-
图 2 X射线照射人体头模的模拟示意图
Figure 2. Simulation diagram of X-ray irradiation on human head model
图 3 四种方法计算大脑吸收剂量对比
Figure 3. Comparison of four methods for calculating brain absorbed dose
图 5 能量30 keV、60 keV和120 keV光子在头模内的运动径迹
Figure 5. 30 keV、60 keV and 120 keV photon tracks in the head phantom
图 6 径迹长度能量沉积法和脉冲高度计数法在水球中的吸收剂量相对误差变化曲线
Figure 6. Curve of relative error of absorbed dose for track length energy deposition method and pulse height method in water sphere
表 1 钯－100源产生的X射线的能量与相对强度
Table 1. Energy and relative intensity of X-ray generated by palladium 100 source
energy/keV relative intensity energy/keV relative intensity 32.66 4.90 86.37 0.05 42.08 13.50 119.18 0.13 53.52 0.08 126.15 15.00 61.60 0.51 139.92 0.35 72.52 0.15 151.88 0.61 74.78 92.00 154.00 0.06 84.00 100.00 158.87 3.20 
下载: 导出CSV
-
[1] 张文仲. 电离辐射粒子在人体组织中能量沉积的微剂量学研究[D]. 北京: 中国人民解放军军事医学科学院, 2003Zhang Wenzhong. Microdosimetry study on energy deposition of ionizing radiation particles in human tissues [D]. Academy of Military Medical Sciences, 2003 [2] 管永红, 黄娇凤, 刘进, 等. 蒙特卡罗技术在放射诊断剂量快速计算中的应用[J]. 强激光与粒子束, 2013, 25(1):193-195 doi: 10.3788/HPLPB20132501.0193Guan Yonghong, Huang Jiaofeng, Liu Jin, et al. Application of Monte Carlo technology to fast dose calculation of radiation therapy[J]. High Power Laser and Particle Beams, 2013, 25(1): 193-195 doi: 10.3788/HPLPB20132501.0193 [3] 宋婷, 周凌宏. 基于蒙特卡罗方法的6 MV Truebeam剂量计算[J]. 强激光与粒子束, 2012, 24(12):2975-2978 doi: 10.3788/HPLPB20122412.2975Song Ting, Zhou Linghong. Dose calculation of 6 MV Truebeam using Monte Carlo method[J]. High Power Laser and Particle Beams, 2012, 24(12): 2975-2978 doi: 10.3788/HPLPB20122412.2975 [4] 曹蕾, 张耀锋, 杨扬, 等. MC模拟无卷积全谱转换法测量X, γ辐射剂量[J]. 强激光与粒子束, 2022, 34:026005 doi: 10.11884/HPLPB202234.210300Cao Lei, Zhang Yaofeng, Yang Yang, et al. Measurement of environmental level X, γ dose with conversion of complete spectra without deconvolution method of MC simulation[J]. High Power Laser and Particle Beams, 2022, 34: 026005 doi: 10.11884/HPLPB202234.210300 [5] Xu X G. An exponential growth of computational phantom research in radiation protection, imaging, and radiotherapy: a review of the fifty-year history[J]. Physics in Medicine and Biology, 2014, 59(18): R233-R302. doi: 10.1088/0031-9155/59/18/R233 [6] 张玲玉, 李瑞, 李刚, 等. γ射线探测器响应函数的JMCT模拟计算[J]. 强激光与粒子束, 2017, 29:016024 doi: 10.11884/HPLPB201729.160454Zhang Lingyu, Li Rui, Li Gang, et al. JMCT simulation of response functions for gamma-ray detectors[J]. High Power Laser and Particle Beams, 2017, 29: 016024 doi: 10.11884/HPLPB201729.160454 [7] 邱有恒, 应阳君, 王敏, 等. 光子能量沉积计算的一种新方法[J]. 原子核物理评论, 2011, 28(1):97-102 doi: 10.11804/NuclPhysRev.28.01.097Qiu Youheng, Ying Yangjun, Wang Min, et al. A new calculation method for energy deposition of photon[J]. Nuclear Physics Review, 2011, 28(1): 97-102 doi: 10.11804/NuclPhysRev.28.01.097 [8] 林辉, 吴宜灿, 陈义学. 基于临床实例的影响蒙特卡罗程序MCNP计算精度和速度的若干参数模拟研究[J]. 原子核物理评论, 2006, 23(2):237-241 doi: 10.3969/j.issn.1007-4627.2006.02.035Lin Hui, Wu Yican, Chen Yixue. Investigation of parameters influencing on simulation precision and speed of Monte Carlo MCNP based on a clinic case[J]. Nuclear Physics Review, 2006, 23(2): 237-241 doi: 10.3969/j.issn.1007-4627.2006.02.035 [9] 赵攀, 陈义学, 林辉, 等. MCNP/MCNPX几何栅元划分方法对精确放疗剂量计算的影响研究[J]. 原子核物理评论, 2006, 23(2):258-262 doi: 10.3969/j.issn.1007-4627.2006.02.040Zhao Pan, Chen Yixue, Lin Hui, et al. Effect of different voxel-uniting methods on the dose calculation of MCNP/MCNPX[J]. Nuclear Physics Review, 2006, 23(2): 258-262 doi: 10.3969/j.issn.1007-4627.2006.02.040 [10] 龚军军, 黄固, 夏文明, 等. 水中浸没γ外照射剂量率转换系数计算方法研究[J]. 辐射防护, 2023, 43(5):460-466Gong Junjun, Huang Gu, Xia Wenming, et al. Study on calculation method of dose rate conversion coefficients for water immersion γ external exposure[J]. Radiation Protection, 2023, 43(5): 460-466 [11] Kim H, Kim J, Hwang J, et al. Pixel-grouping G(E) functions for estimating dose rates from unknown source distributions with a position-sensitive detector[J]. Sensors, 2023, 23: 4591. doi: 10.3390/s23104591 [12] 祝美英, 袁成前, 赵家强. 基于点核法的γ辐射剂量计算软件设计[J]. 核电子学与探测技术, 2022, 42(5):823-827Zhu Meiying, Yuan Chengqian, Zhao Jiaqiang. Design of gamma radiation dose calculation software based on point kernel method[J]. Nuclear Electronics & Detection Technology, 2022, 42(5): 823-827 [13] 丁逸仙. 基于人体模型的中子通量—剂量转换系数模拟研究[D]. 成都: 成都理工大学, 2014Ding Yixian. Neutron flux to dose coefficient simulate research based on phantom[D]. Chengdu: Chengdu University of Technology, 2014 [14] 邱有恒, 应阳君, 王敏, 等. 提高光子能量沉积计算效率的几种技巧[J]. 原子核物理评论, 2011, 28(2):204-208 doi: 10.11804/NuclPhysRev.28.02.204Qiu Youheng, Ying Yangjun, Wang Min, et al. Several techniques on increasing computation efficiency of photon energy deposition[J]. Nuclear Physics Review, 2011, 28(2): 204-208 doi: 10.11804/NuclPhysRev.28.02.204 [15] 鱼红亮, 郑传城, 孙亮. MCNP计算含肿瘤Snyder修正头部模型的硼中子俘获治疗剂量[J]. 原子能科学技术, 2010, 44(1):89-94 doi: 10.7538/yzk.2010.44.01.0089Yu Hongliang, Zheng Chuancheng, Sun Liang. Boron neutron capture therapy dose calculation for tumor modified Snyder head phantom using MCNP[J]. Atomic Energy Science and Technology, 2010, 44(1): 89-94 doi: 10.7538/yzk.2010.44.01.0089 [16] Goorley J T, Kiger W S, Zamenhof R G. Reference dosimetry calculations for neutron capture therapy with comparison of analytical and voxel models[J]. Medical Physics, 2002, 29(2): 145-156. doi: 10.1118/1.1428758 [17] ICRP. Conversion coefficients for use in radiological protection against external radiation[R]. ICRP Publication 74, 1996. [18] ICRP. Conversion coefficients for radiological protection quantities for external radiation exposures[R]. ICRP Publication 116, 2010. [19] Nuclear Energy Agency. Computing radiation dosimetry--CRD 2002[C]. Sacavém: Nuclear Energy Agency, 2002. [20] 程梦云, 王文, 范言昌, 等. 基于中国辐射虚拟人Rad-HUMAN的中子剂量转换系数及分析[J]. 原子能科学技术, 2015, 49(s1):22-29 doi: 10.7538/yzk.2015.49.S0.0022Cheng Mengyun, Wang Wen, Fan Yanchang, et al. Neutron dose conversion coefficient based on Chinese female computational phantom rad-HUMAN[J]. Atomic Energy Science and Technology, 2015, 49(s1): 22-29 doi: 10.7538/yzk.2015.49.S0.0022 -
点击查看大图
图(7) / 表(1)
计量
- 文章访问数: 51
- HTML全文浏览量: 30
- PDF下载量: 3
- 被引次数: 0
