Coded-aperture image reconstruction algorithm based on maximum a posteriori estimation
-
摘要: 图像重建算法对编码孔伽马相机的成像性能有重要的影响，然而广泛使用的最大似然期望最大化（MLEM）算法无法在较强干扰背景下有效抑制图像中的噪声，当超过一定迭代次数后，图像信噪比会逐渐降低。针对MLEM算法的这一“病态性”问题开展了研究。首先将最大后验估计（MAP）算法应用于编码孔图像重建，接着分析了算法中Gibbs先验函数的邻域大小和权值系数等关键参数的选取方法。然后使用编码孔相机开展了成像实验，对比了MLEM算法与MAP算法对22Na点源的图像重建结果。结果表明，在300～
1200 次迭代下，MLEM重建图像中出现了明显的噪点，且随着迭代深入图像质量逐渐变差；而MAP重建图像没有出现明显噪点，重建图像的平均梯度相较于MLEM降低了26.45%～49.16%，对比度噪声比（CNR）提升了42.32%～351.07%。另外，对比了3×3和5×5邻域大小时的多点源图像重建结果，结果显示，邻域过小会导致重建图像的热点亮度降低，与理论分析结果一致。最后，分别对比了MLEM与MAP算法在较远距离和较强干扰两种场景下的成像结果，MAP算法均表现出更好的信噪比性能。-
关键词:
- 编码孔成像 /
- 最大似然期望最大化算法 /
- 最大后验估计 /
- 贝叶斯定理 /
- 马尔科夫随机场
Abstract: Image reconstruction algorithms significantly influence the imaging performance of coded-aperture gamma cameras. However, the widely used Maximum Likelihood Expectation Maximization (MLEM) algorithm falls short in effectively suppressing noise amidst stronger background interference because it relies on the system response matrix under ideal conditions. This paper presents corresponding research and improvements regarding the “pathological” nature of the MLEM algorithm. Firstly, the maximum a posteriori (MAP) algorithm was applied to the image reconstruction of coded aperture imaging, followed by an analysis of the selection methods for key parameters such as the neighborhood size and weight coefficient within the Gibbs prior function of the algorithm. Then, we conducted imaging experiments using the prototype of the coded-aperture gamma camera and compared the image reconstruction results of the MLEM algorithm and the MAP algorithm for the 22Na point source. In the range of 300 to1200 iterations, the MLEM reconstructed images exhibited noticeable noise spots, with image quality progressively deteriorating as the iterations deepened. In contrast, the MAP reconstructed images did not present any significant noise spots. The average gradient of the reconstructed images was reduced by 26.45% to 49.16% compared to MLEM, and the contrast-to-noise ratio (CNR) was improved by 42.32% to 351.07%. Furthermore, we compared the reconstruction results of multi-point source images with 3 × 3 and 5 × 5 neighborhood sizes. The results indicate that smaller neighborhood size leads to a decrease in the brightness of the hotspots in the reconstructed images, consistent with the theoretical analysis. Finally, we compared the imaging results of the MLEM and MAP algorithms in two separate scenarios: one with greater distance and the other with stronger interference. In both scenarios, the MAP algorithm demonstrated better signal-to-noise ratio (SNR) performance. -
图 2 3倍采样数时的点扩散函数和势函数的邻域权值系数
Figure 2. Point spread function and neighbourhood weight coefficients of the potential function at 3 times the samples
图 3 邻域范围过小会导致热点值降低
Figure 3. Too small a neighbourhood range results in lower hotspot values
图 4 惩罚系数计算方法示意图
Figure 4. Schematic diagram of the penalty coefficient calculation method
图 5 编码孔伽马相机示意图和实物图
Figure 5. Schematic and photograph of the coded-aperture gamma camera
图 9 22Na点源在逐渐递增迭代次数下的MLEM和MAP重建图像
Figure 9. MLEM and MAP reconstruction images of 22Na point source with increasing iterations
图 10 22Na点源在逐渐递增迭代次数下的MLEM与MAP重建图像二维切片
Figure 10. 2D slices of MLEM and MAP reconstructed images for a 22Na point source with increasing iterations
图 11 在300、500、800和
1200 迭代次数下，MLEM和MAP算法重建图像的平均梯度值与CNR值对比Figure 11. Comparison of the mean gradient values and CNRs of reconstructed images using MLEM and MAP algorithms at 300, 500, 800, and
1200 iterations
图 12 相同参数（δ=0.003，β=0.03，迭代500次）下，使用MLEM、3×3邻域MAP和5×5邻域MAP算法的多点22Na源重建结果对比
Figure 12. Comparison of the reconstruction results of multipoint 22Na sources using MLEM, 3×3 neighbourhood MAP and 5×5 neighbourhood MAP algorithms with the same parameters (δ = 0.003, β = 0.03, 500 iterations)
图 13 对同一个辐射源，MLEM重建图像和分别使用L1、L2、Huber势函数时的MAP重建图像二维切片
Figure 13. 2D slices of the MLEM reconstructed image and the MAP reconstructed image when using the L1, L2, and Huber potential functions, respectively, for the same radiation source
图 14 远距离实景成像时，MLEM与MAP算法的成像效果对比
Figure 14. Comparison of the effectiveness between the MLEM and the MAP algorithms when imaging at long distances
-
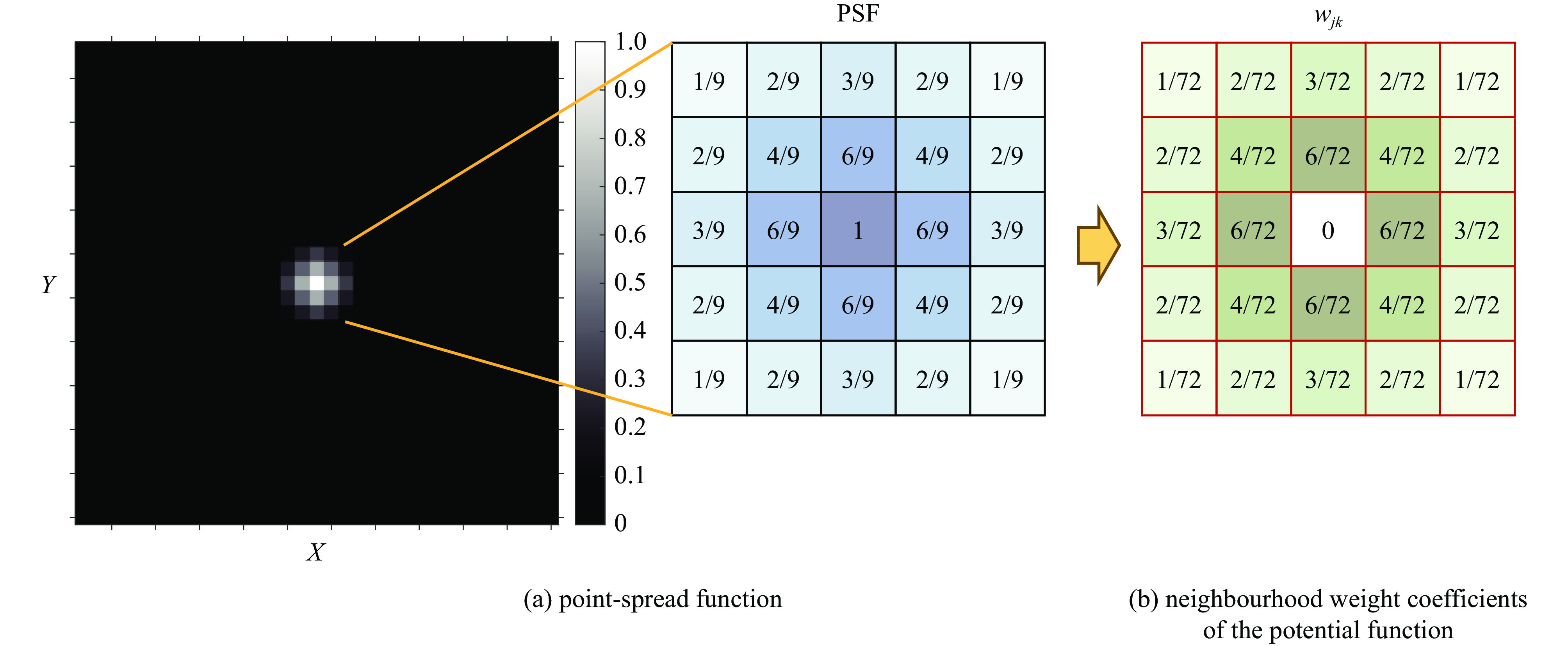
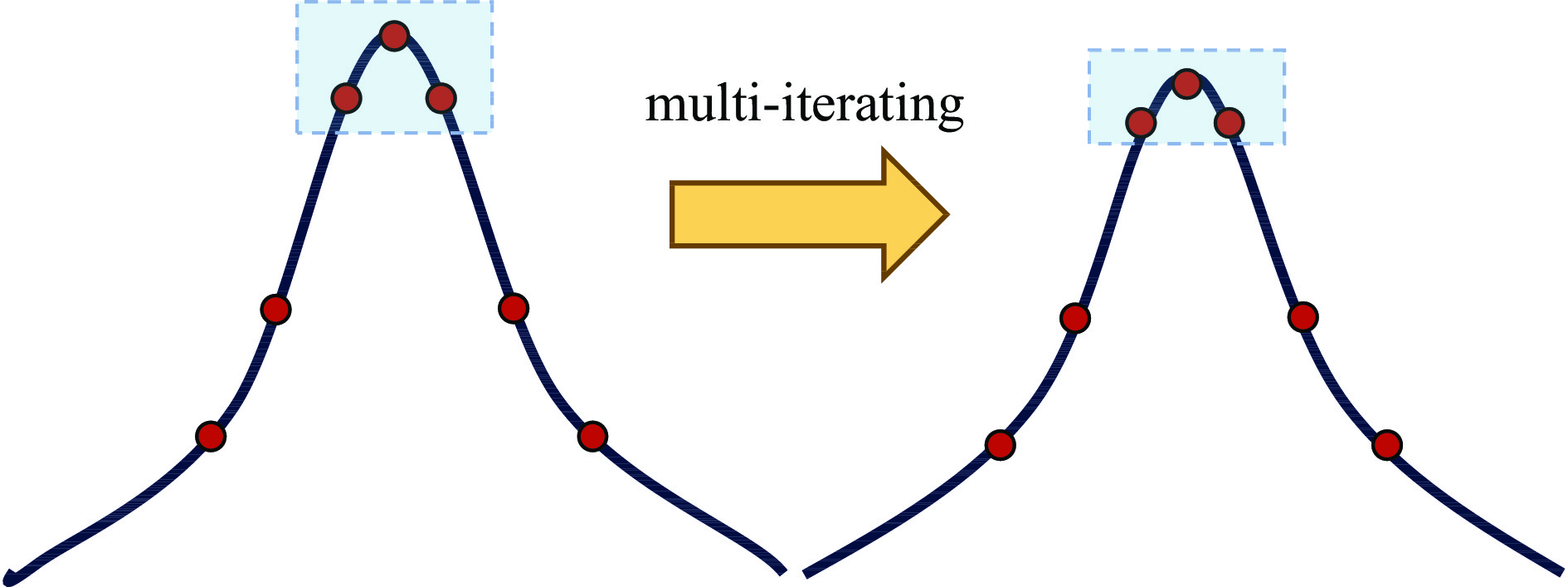
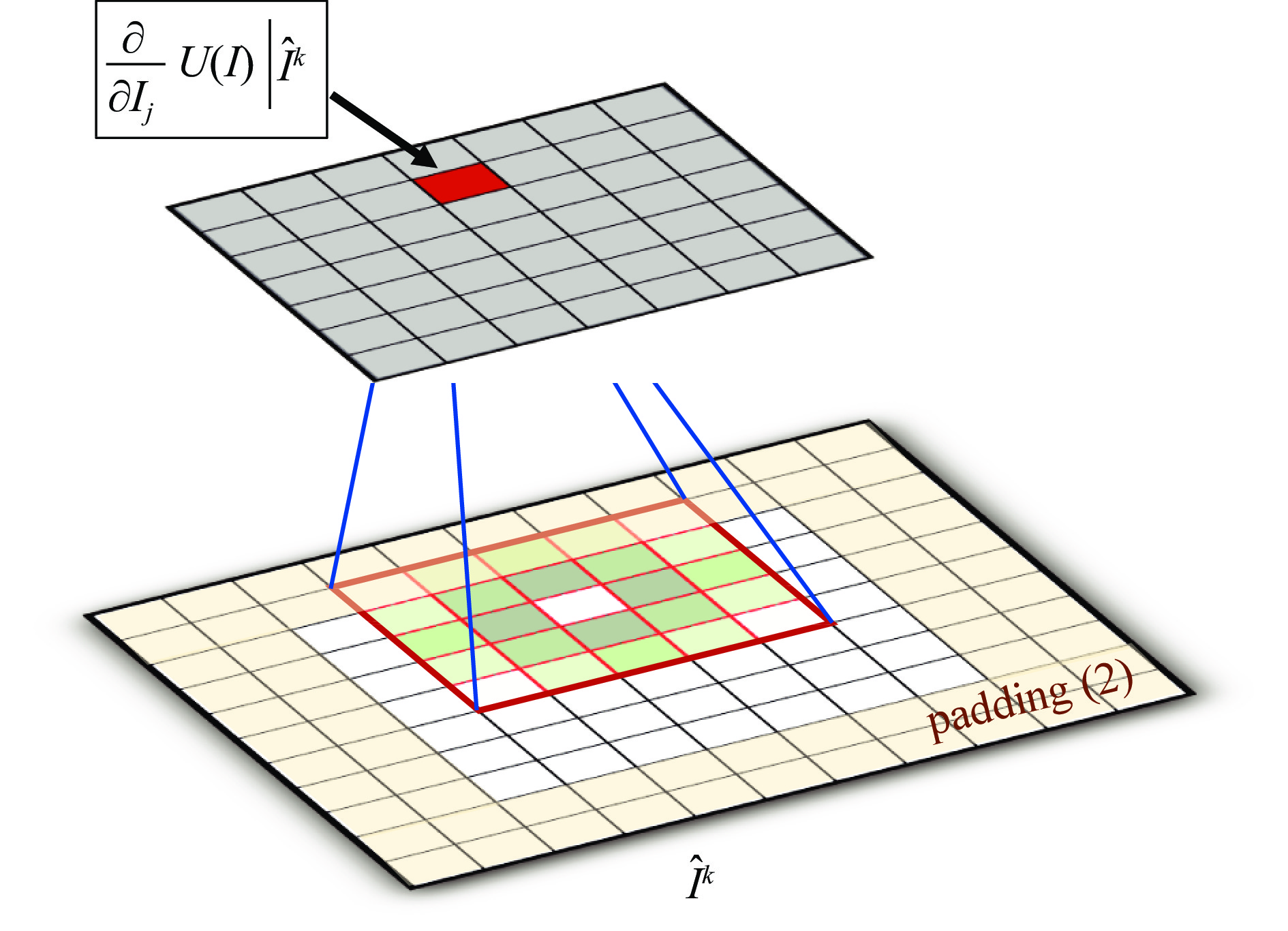
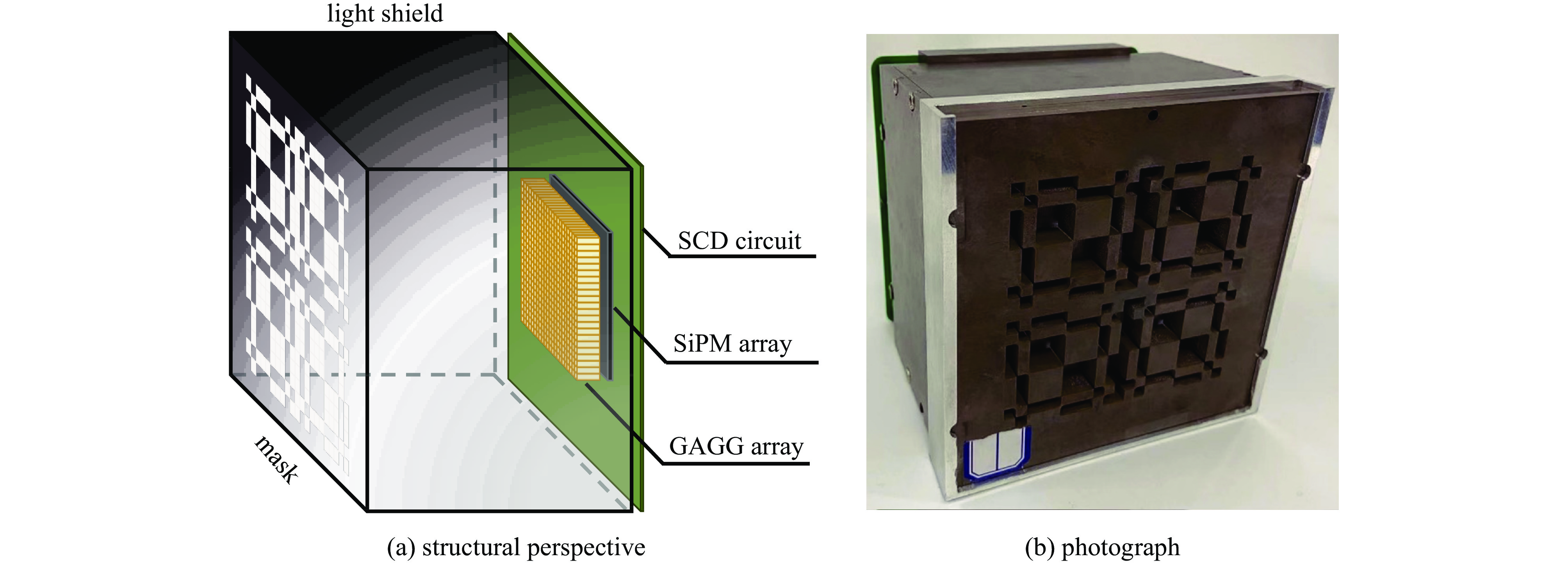
[1] 昂文胜, 董顺成, 杜永欢, 等. 编码孔径辐射成像定位技术[J]. 辐射研究与辐射工艺学报, 2024, 42: 020801Ang Wensheng, Dong Shuncheng, Du Yonghuan, et al. Positioning technique of coded aperture radiation imaging[J]. Journal of Radiation Research and Radiation Processing, 2019, 42: 020801 [2] 江少恩, 刘忠礼, 郑志坚. 惯性约束聚变实验中编码成像的反投影解码[J]. 强激光与粒子束, 1999, 11(2):210-214Jiang Shaoen, Liu Zhongli, Zheng Zhijian. Decoding using back-project algorithm from coded Image in ICF[J]. High Power Laser and Particle Beams, 1999, 11(2): 210-214 [3] 王蒙蒙. 编码孔径成像技术[J]. 中国新技术新产品, 2011(4):59 doi: 10.3969/j.issn.1673-9957.2011.04.058Wang Mengmeng. Coded aperture imaging technology[J]. China New Technology and New Products Magazine, 2011(4): 59 doi: 10.3969/j.issn.1673-9957.2011.04.058 [4] Carrel F, Khalil R A, Colas S, et al. GAMPIX: A new gamma imaging system for radiological safety and Homeland Security Purposes[C]//2011 IEEE Nuclear Science Symposium Conference Record. 2011: 4739-4744. [5] Liu Bin, Lv Huanwen, Xu Hu, et al. A novel coded aperture for γ-ray imaging based on compressed sensing[J]. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment, 2022, 1021: 165959. doi: 10.1016/j.nima.2021.165959 [6] Jeong M, Hammig M D. Comparison of gamma ray localization using system matrixes obtained by either MCNP simulations or ray-driven calculations for a coded-aperture imaging system[J]. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment, 2020, 954: 161353. doi: 10.1016/j.nima.2018.10.031 [7] 张锐. 基于人工神经网络的编码孔γ相机成像方法研究[D]. 南京: 南京航空航天大学, 2020Zhang Rui. Research on coded aperture gamma camera imaging method based on artificial neural network[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2020 [8] 张斌, 王英, 艾宪芸, 等. 基于约束的MLEM图像重建算法[C]//北京核学会第十届(2014年)核应用技术学术交流会论文集. 2014Zhang Bin, Wang Ying, Ai Xianyun, et al. Constraint-based MLEM image reconstruction algorithm[C]//Beijing Nuclear Society 10th (2014) Nuclear Application Technology Symposium. 2014 [9] 李汉平, 王锋, 艾宪芸. 编码板成像系统MLEM算法优化[J]. 核技术, 2017, 40:020404 doi: 10.11889/j.0253-3219.2017.hjs.40.020404Li Hanping, Wang Feng, Ai Xianyun. Algorithm optimization of MLEM in coded aperture imaging system[J]. Nuclear Techniques, 2017, 40: 020404 doi: 10.11889/j.0253-3219.2017.hjs.40.020404 [10] 侯英伟, 宋玉收, 孙世杰, 等. 编码成像MLEM互补算法的参数优化[J]. 哈尔滨工程大学学报, 2021, 42(12):1799-1804 doi: 10.11990/jheu.202107069Hou Yingwei, Song Yushou, Sun Shijie, et al. Parameter optimization of coded aperture imaging based on the MLEM complementary algorithm[J]. Journal of Harbin Engineering University, 2021, 42(12): 1799-1804 doi: 10.11990/jheu.202107069 [11] Lee T, Lee W. High performance γ-ray imager using dual anti-mask method for the investigation of high-energy nuclear materials[J]. Nuclear Engineering and Technology, 2021, 53(7): 2371-2376. doi: 10.1016/j.net.2021.01.027 [12] 刘祎, 桂志国, 张权, 等. 一种基于指数型先验分布的正电子发射断层图像重建算法[J]. 中国组织工程研究与临床康复, 2010, 14(52):9760-9763Liu Yi, Gui Zhiguo, Zhang Quan, et al. Positron emission tomography image reconstruction algorithm based on an exponential Markov random field prior model[J]. Journal of Clinical Rehabilitative Tissue Engineering Research, 2010, 14(52): 9760-9763 [13] 管永红, 王鹏来, 景越峰. 基于贝叶斯准则的闪光照相图像重建[J]. 强激光与粒子束, 2011, 23(9):2495-2498 doi: 10.3788/HPLPB20112309.2495Guan Yonghong, Wang Penglai, Jing Yuefeng. Reconstruction of flash radiographic image based on Bayesian approach[J]. High Power Laser and Particle Beams, 2011, 23(9): 2495-2498 doi: 10.3788/HPLPB20112309.2495 [14] Dempster A P, Laird N M, Rubin D B. Maximum likelihood from incomplete data via the EM algorithm[J]. Journal of the Royal Statistical Society: Series B (Methodological), 1977, 39(1): 1-22. doi: 10.1111/j.2517-6161.1977.tb01600.x [15] Shepp L A, Vardi Y. Maximum likelihood reconstruction for emission tomography[J]. IEEE Transactions on Medical Imaging, 1982, 1(2): 113-122. doi: 10.1109/TMI.1982.4307558 [16] Lange K, Carson R. EM reconstruction algorithms for emission and transmission tomography[J]. Journal of Computer Assisted Tomography, 1984, 8(2): 306-316. [17] 陈伯显, 张智. 核辐射物理及探测学[M]. 哈尔滨: 哈尔滨工程大学出版社, 2011Chen Boxian, Zhang Zhi. Nuclear radiation physics and detection[M]. Harbin: Harbin Engineering University Press, 2011 [18] 何骞. 基于最大后验概率的PET图像重建算法研究[D]. 长沙: 湖南大学, 2016He Qian. The research of PET image reconstruction algorithm based on maximum a posterior[D]. Changsha: Hunan University, 2016 [19] Levitan E, Herman G T. A maximum a posteriori probability expectation maximization algorithm for image reconstruction in emission tomography[J]. IEEE Transactions on Medical Imaging, 1987, 6(3): 185-192. doi: 10.1109/TMI.1987.4307826 [20] 苏明. 基于MRF先验模型的图像超分辨率重建算法研究[D]. 合肥: 中国科学技术大学, 2011Su Ming. Research for super-resolution image reconstruction algorithm based on MRF prior model[D]. Hefei: University of Science and Technology of China, 2011 [21] Rabiner L R. A tutorial on hidden Markov models and selected applications in speech recognition[J]. Proceedings of the IEEE, 1989, 77(2): 257-286. doi: 10.1109/5.18626 [22] Besag J. Spatial interaction and the statistical analysis of lattice systems[J]. Journal of the Royal Statistical Society: Series B (Methodological), 1974, 36(2): 192-225. doi: 10.1111/j.2517-6161.1974.tb00999.x [23] Tsallis C. Possible generalization of Boltzmann-Gibbs statistics[J]. Journal of Statistical Physics, 1988, 52(1/2): 479-487. [24] Geman S, Geman D. Stochastic relaxation, Gibbs distributions, and the Bayesian restoration of images[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 1984, PAMI-6(6): 721-741. doi: 10.1109/TPAMI.1984.4767596 [25] Besag J. Towards Bayesian image analysis[J]. Journal of Applied Statistics, 1993, 20(5/6): 107-119. [26] Mumcuoglu E Ü, Leahy R M, Cherry S R. Bayesian reconstruction of PET images: methodology and performance analysis[J]. Physics in Medicine & Biology, 1996, 41(9): 1777-1807. [27] Green P J. On use of the EM algorithm for penalized likelihood estimation[J]. Journal of the Royal Statistical Society: Series B (Methodological), 1990, 52(3): 443-452. doi: 10.1111/j.2517-6161.1990.tb01798.x [28] Gottesman S R, Fenimore E E. New family of binary arrays for coded aperture imaging[J]. Applied Optics, 1989, 28(20): 4344-4352. doi: 10.1364/AO.28.004344 [29] Agostinelli S, Allison J, Amako K, et al. GEANT4—a simulation toolkit[J]. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment, 2003, 506(3): 250-303. [30] Allison J, Amako K, Apostolakis J, et al. Geant4 developments and applications[J]. IEEE Transactions on Nuclear Science, 2006, 53(1): 270-278. doi: 10.1109/TNS.2006.869826 [31] 龚胡, 石强. 基于平均梯度的边缘保持指数的图像质量评价[C]//2011年信息技术、服务科学与工程管理国际学术会议论文集. 2011Gong Hu, Shi Qiang. Image quality assessment based on the mean gradient of edge preserve index[C]//Proceedings of the 2011 International Conference on Information, Services and Management Engineering. 2011 [32] 吴鹃. 基于自适应调节下信息特征惩罚的图像修复算法[J]. 国外电子测量技术, 2019, 38(6):33-37Wu Juan. Study on image inpainting based on information feature penalty with adaptive adjustment[J]. Foreign Electronic Measurement Technology, 2019, 38(6): 33-37 [33] Zhu Balin, Wang Zhonghai, Gao Tai, et al. A new contrast-to-noise ratio for image quality characterization of a coded-aperture γ camera[J]. Applied Radiation and Isotopes, 2021, 170: 109592. doi: 10.1016/j.apradiso.2021.109592 -
下载:
点击查看大图
计量
- 文章访问数: 215
- HTML全文浏览量: 67
- PDF下载量: 30
- 被引次数: 0
